Time Value of Money
TL;DR
The time value of money is the concept that quantifies how money received today is preferred over an identical sum at a later point in time. It is a foundational theory in the study of finance. The two key elements to determining the time value of money are:
-
Interest Rate
The amount of money that can be earned during each period. -
Time Horizon
The number of periods before the money is returned.
Microsoft Excel offers a financial function PV to easily calculate the present value of an amount of money given some
future amount. Similarly, the function FV calculates the future value of a sum given a constant interest rate and someone
number of periods.
A Dollar Today...
is worth more than a dollar tomorrow. That is the gist behind the time value of money, and it is one of the most important and foundational concepts in the study of finance.
Suppose you discovered a way to grow money on trees, but it took 500 years for the trees to mature, how would you monetize this invention so that you can enjoy the “fruits” of your labor without waiting 500 years? What is an appropriate price to pay for your secret? Having a good understanding of the growth and time value of money is crucial to basic financial literacy, but also important to learning intermediate models of valuation like discounted cash flow analysis (DCF). Let's also throw in the fact that you are Duncan MacLeod of the Clan MacLeod, an immortal being who happens to also fancy the finer things in life.
Let’s make the money tree example a bit more concrete. Let’s say that in 500 years, your trees will make you a millionaire by sprouting 1 million dollar bills. Assuming no other catastrophe befalls your tree grove, how much is that $1,000,000 worth in today’s dollars? In other words, how much money are you willing to give up to get paid today versus 500 years from now. To solidify this example, let’s also say that you can put your money in the bank, and have a guaranteed 5% interest each year.
Given these parameters, if we put $1,000 in the bank today, that money would grow to $10,500 by this time next year. More concretely, we take today’s sum and multiply by (1 + r), where r is the interest rate of the bank.
Year One:
Present Value x (1 + Interest Rate) = Value One Period In the Future
$1,000 x (1 + 0.05) = $1,050
In year two, we take the value from year one and again multiply THAT by (1 + r):
Year Two:
Present Value x (1 + Interest Rate) x (1 + Interest Rate) = Value Two Periods In the Future
Present Value x (1 + Interest Rate)2 = Value Two Periods In the Future
$1,000 x (1 + 0.05)2 = $1,102.50
The formula becomes clearer at this point, the future value of your money is simply the amount you have today, multiplied by (1 + r) for each year being considered. Extrapolating out to 500 Years, we get the following:
Year Five Hundred:
Present Value x (1 + Interest Rate)500 = Value Five Hundred Periods In the Future
$1,000 x (1 + 0.05)500 = $39,323,261,827,218
THIRTY NINE TRILLION DOLLARS.
This value is much more than what your money tree will yield, so we can say with confidence that putting that money in the bank today is a much better investment than buying a grove of money trees. In fact, we can reverse engineer our formula to see exactly how much we should be paying for those trees. Doing some simple algebra to our original formula:
Present Value Needed to Obtain $1,000,000 in 500 Years
Present Value x (1 + Interest Rate)500 = Value Five Hundred Periods In the Future
Present Value = Value Five Hundred Periods In the Future / (1 + Interest Rate)500
Present Value = 1,000,000 / (1 + 0.05)500
Present Value = $0.0000254
And there you have it. In our example assuming a guaranteed 5% interest at the bank, the present value of a grove of trees that will produce a million one dollar bills 500 years in the future is well under a penny. So while your brilliant invention may look lucrative in theory, the erosion of the time value makes the entire enterprise much less impressive.
Calculating Present Value in Excel
Microsoft Excel is a tool commonly used in financial analysis, especially as it relates to valuation modeling. Unsurprisingly,
Excel offers a financial function PV that facilitates the calculation of present values, assuming a constant rate of interest.
The syntax of the PV function is as follows:
PV(rate, nper, pmt, [fv],[type])
** Brackets Denote Optional Parameters
Let's go through each parameter:
rate
The interest rate for each period. For our example, it was 5% or 0.05.
nper
The number of periods in your calculation. For our example, this number is 500.
pmt
The payment made in each period. This is a value dealing with the repayment of loans. Because this is not pertinent to our example,
we would enter “0”.
fv
The future value obtained. In our example the FV will be $1,000,000.
To find the sub-penny value we calculated earlier, we simply plug in these parameters as illustrated below:
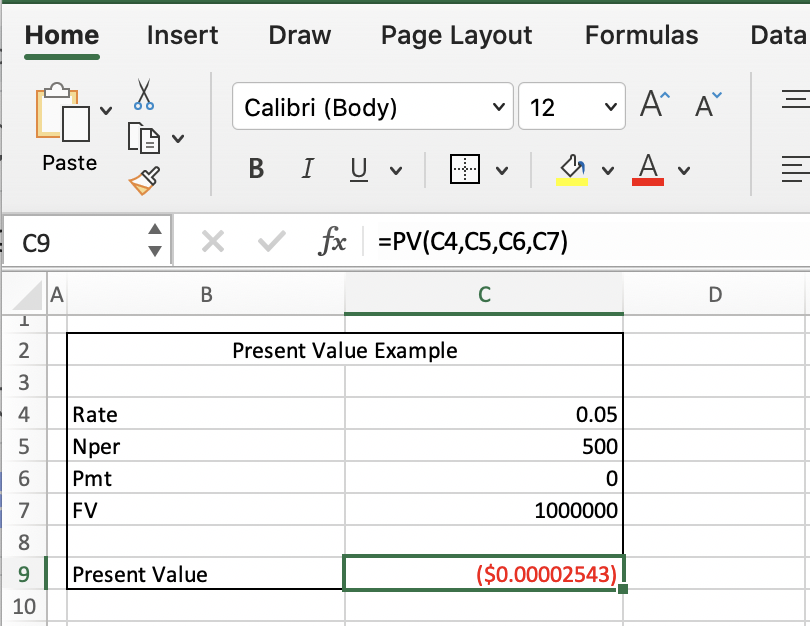
Present Value here is a negative number (always), demonstrating the amount someone has to put in to obtain a future value of $1,000,000.
Now supposed we wanted to find the Future Value of a $1,000 investment yielding 5% over 500 years, we can take advantage of the Excel
function FV, which effectively reverses the formula to calculate the future values.
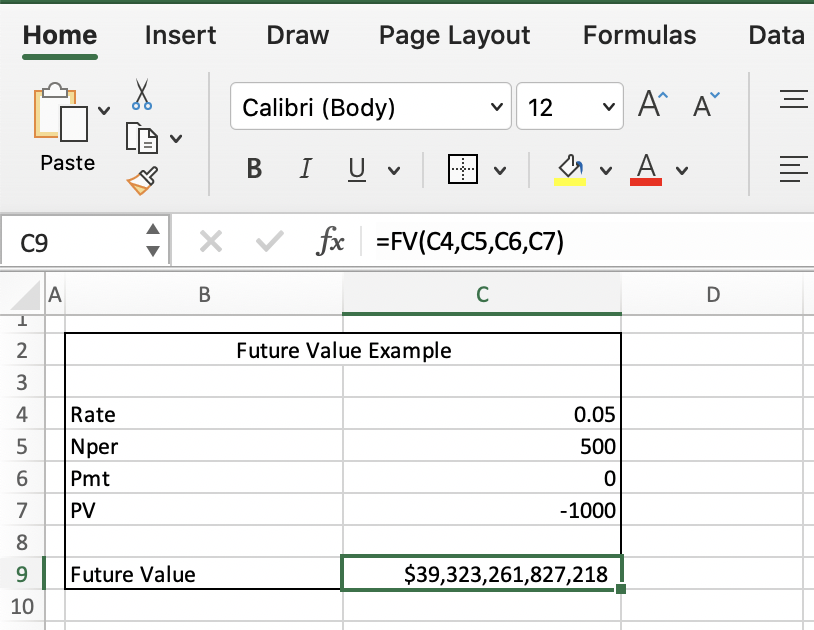
Because the syntax is very much along the same vein as that of Present Value, we will forgo the breakdown and leave that to our astute readers.